A) L(x) = ![]() x +
x + ![]()
B) L(x) = ![]() x +
x + ![]()
C) L(x) = ![]() x +
x + ![]()
D) L(x) = ![]() x +
x + ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the interval of convergence of the series.
-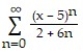
A) 1 < x < 9
B) -1 x 11
C) 4 x < 6
D) -1 < x < 11
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the first four nonzero terms in the Maclaurin series for the function. -f(x) = x sin( 3x)
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the function represented by the power series.
-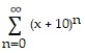
A) ![]()
B)
- ![]()
C) ![]()
D)
- ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the series' radius of convergence.
-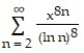
A) , for all x
B) 2
C) 1
D) 0
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the first four terms of the binomial series for the given function.
-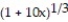
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the first four terms of the binomial series for the given function.
-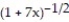
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the first four nonzero terms in the Maclaurin series for the function.
-f(x) = 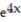
A) 1 + 4x + ![]() +
+ ![]() + . . .
+ . . .
B) 1 + 4x + ![]() +
+ ![]() + . . .
+ . . .
C) x + 4x + ![]() +
+ ![]() + . . .
+ . . .
D) 4x + 64 ![]() + 1024
+ 1024 ![]() + 16,384
+ 16,384 ![]() + . . .
+ . . .
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the series' radius of convergence.
-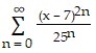
A) 1
B) , for all x
C) 5
D) 10
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the first four terms of the binomial series for the given function.
-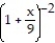
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the Taylor polynomial of order 3 generated by f at a. -f(x) = ln(x + 1) , a = 5
A) ![]() (x) = ln 6 -
(x) = ln 6 - ![]() +
+ ![]() -
- ![]()
B) ![]() (x) = ln 4 -
(x) = ln 4 - ![]() +
+ ![]() -
- ![]()
C) ![]() (x) = ln 4 +
(x) = ln 4 + ![]() +
+ ![]() +
+ ![]()
D) ![]() (x) = ln 6 +
(x) = ln 6 + ![]() -
- ![]() +
+ ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the function represented by the power series.
-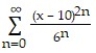
A) ![]()
B) - ![]()
C) ![]()
D) - ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the Taylor series generated by f at x = a.
-f(x) = 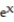 , a = 7
, a = 7
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the linear approximating polynomial for the function centered at a.
-f(x) = 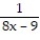 , a = 0
, a = 0
A) L(x) = ![]() x +
x + ![]()
B) L(x) = - ![]() x +
x + ![]()
C) L(x) = ![]() x -
x - ![]()
D) L(x) = - ![]() x -
x - ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the Taylor series generated by f at x = a.
-f(x) = 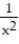 , a = 7
, a = 7
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the first four terms of the binomial series for the given function.
-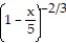
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use power series operations to find the Taylor series at x = 0 for the given function.
-f(x) = 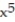
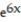
A) ![]()
B) ![]()
C) ![]()
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the interval of convergence of the series.
-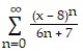
A) x < 9
B) 7 x < 9
C) 2 x < 14
D) 7 < x < 9
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Use Taylor series to evaluate the limit.
-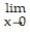
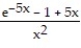
A) ![]()
B) 25
C) 0
D) ![]()
Correct Answer

verified
Correct Answer
verified
Multiple Choice
Find the series' radius of convergence.
-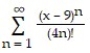
A) 2
B) 0
C) , for all x
D) 1
Correct Answer

verified
Correct Answer
verified
Showing 61 - 80 of 103
Related Exams